On Double Counterpoint
Second Section
Conclusion
All these examples suggest an important remark; which is, that notwithstanding the denominations of triple and quadruple counterpoint at the tenth, or at the twelfth, there is no true triple or quadruple counterpoint save that of the octave.
And in fact, the combinations of this kind of counterpoint alone permit the composition of a piece, in three or in four voices (or even in a greater number of voices), in which the parts can admit of complete inversion; in a good quadruple counterpoint at the octave, the parts can, without difficulty, change places, and supple a multitude of fresh aspects, by being transposed to the upper, the middle, or the lower part, while the lower ascends from the middle to the upper part.
But it is – so to speak – impossible to compose in three or in four voices, upon condition that each of the parts may, in its turn, be transposed to the third (or tenth) above or below, to the fifth (or twelfth), above or below, without ceasing to be in harmony with these three other parts. It is therefore necessary to use some ingenuity for the obtaining of so-called triple and quadruple counterpoints at the tenth and at the twelfth.
In composing – as has been said – a double counterpoint in one or other of these intervals, by contrary or oblique movement, so as never to have two successive thirds, and avoiding all prepared discords, it is possible to add to each of these two parts another part in thirds, and the counterpoint becomes triple or quadruple, by the addition of one of these two parts, or both at a time.
But in quadruple counterpoint at the tenth, obtained by this measure, an inversion at the tenth is no longer possible; since it is the inversions themselves which – proceeding with the principal parts – are to form the four parts: but this counterpoint can be inverted at the octave; that is to say, it is possible to change the places occupied by the different parts, if care has been taken to observe the rules of double counterpoint at the octave.
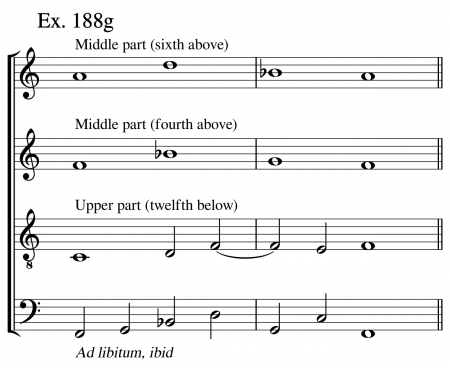
Quadruple counterpoint at the twelfth is more real and more varied: that is to say, among the four parts thus combined, there will be always two which may actually be transposed:
- the one a fifth above,
- the other a fifth below.
These two are the two principal parts, which on that account are not the less able to proceed in thirds with the two added parts.
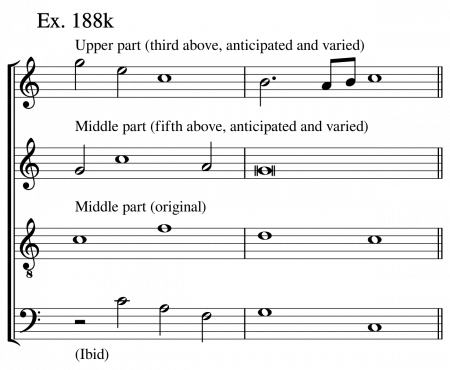
Before concluding this section, a series of examples will be given from the learned Padre Martini, relative to these counterpoints; in which will be seen the employment and the use that should be made of them.
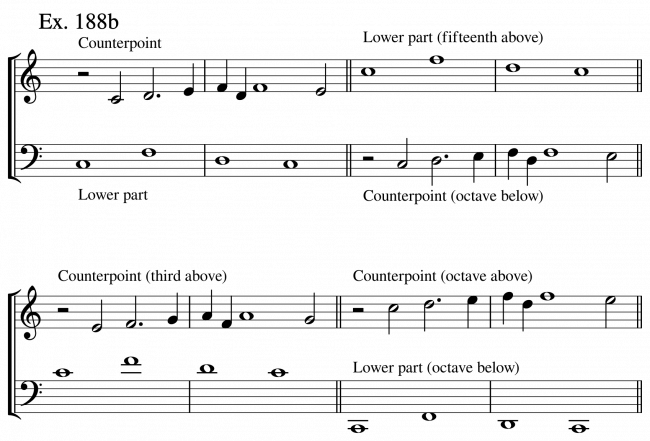
1st order
2nd order
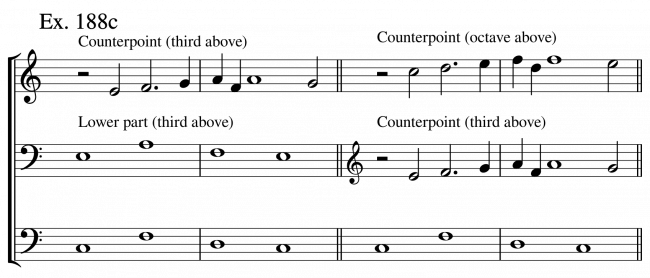
2nd order, continued
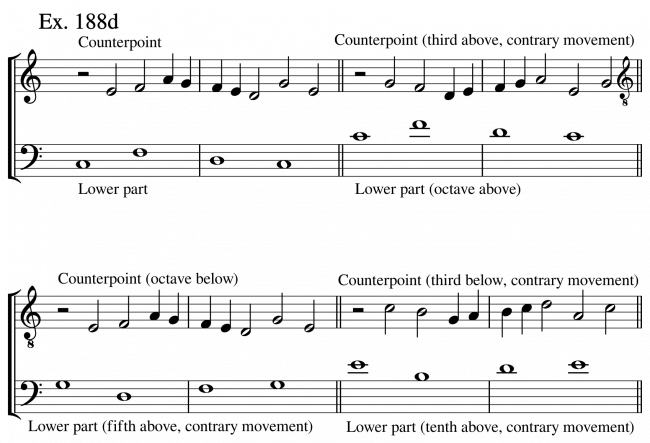
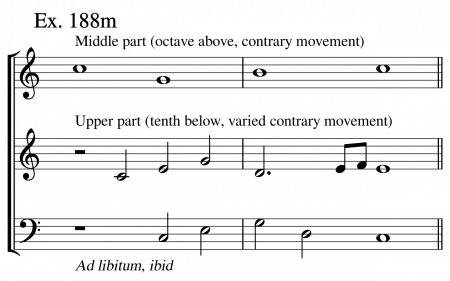
3rd order: Contrary movements
3rd order: Contrary movements, continued
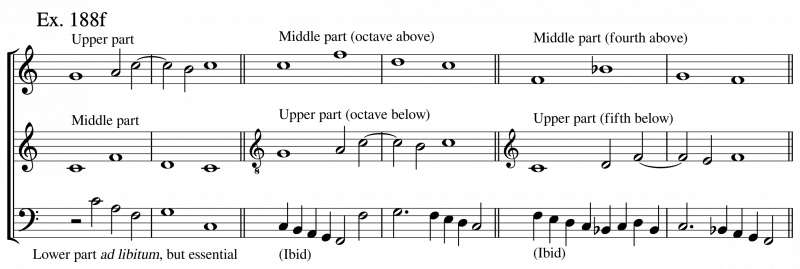
4th order
4th order, continued
4th order, continued
5th order, several examples