Two-Part Counterpoint
Two-part counterpoint is the more strict, both in the ancient and the modern system. The reason for this is plain: the fewer the difficulties to be vanquished, the more the rules must be severe. Two-part writing does not involve so many trammels, as a larger number of parts progressing together; so that the strictness of this kind of composition diminishes in proportion as the number of parts increase.
First Order: Note Against Note
Rule 1
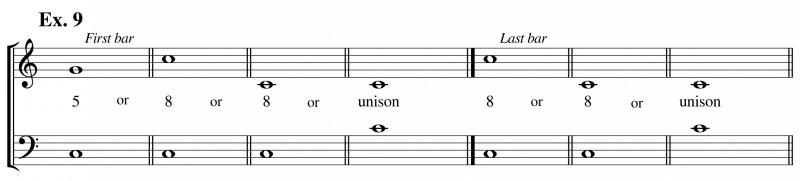
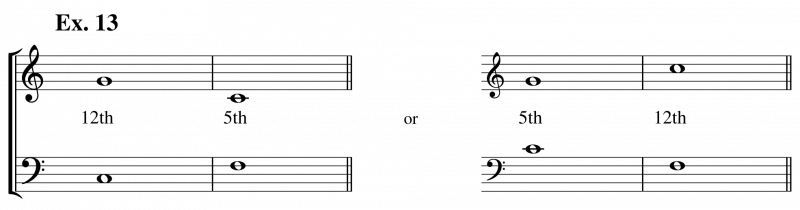
The commencement 1) must be a perfect concord 2), and the termination 3) also, so that the first bar may be either a fifth, an octave (or a unison), and the last bar should be simply an octave (or a unison). Let it be borne in mind, once for all, that by the word “fifth” is also understood the twelfth; and by the word “octave”, the fifteenth, according to the relative distances of the voices employed; and the same will apply to all intervals which may be doubled or tripled.
Rule 2
The parts should progress always by concords 4), with endeavour to avoid the unison, excepting at the first or last bar.
Observation – The principal aim in counterpoint being to produce harmony, unison is forbidden, because it produces none. This does not hold good with regard to the octave; for, although the octave is almost in the same condition with the unison, yet the difference which exists between the grave and acute sound renders it less devoid of harmony than the unison.
Rule 3
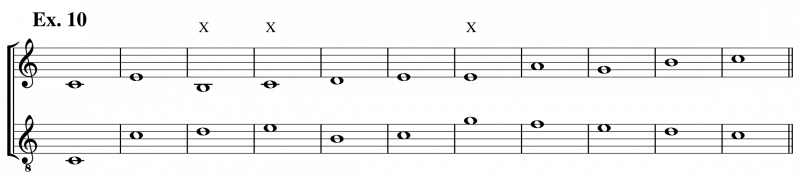
It is sometimes admissible to let the higher part pass beneath the lower part, always, however, taking care that they shall be in concord 5), and not allowing this method to continue too long, as it is only admissible in case of extremity, or in order to make the parts flow well, since the pupil should, as we have just said, write for voices:–
These marks “X” indicate the places where the higher part passes beneath the lower. It cannot, however, too strongly be recommended, that this method should never be employed but with great reserve.
Rule 4
Several perfect concords 6) of the same denomination should never be permitted to succeed each other, at whatever pitch they may occur; consequently, two fifths and two octaves in succession are prohibited.
This prohibition is applicable to every kind of strict composition, in two parts, as well as in more.
Observation – A succession of octaves renders harmony well-nigh void; a succession of fifths forms a discordance, because the upper part progresses in one key, at the same time that the lower part progresses in another. For example, if, in the key of C, an upper part be added, which gives a perfect fifth at each bar, thus–
Parallel fifths by conjunct motion
It follows, that one part would be in C, while the other would be in G. It is from this concurrence of two keys, that the discordance arises, and consequently, the prohibition to introduce several fifths in succession; as, even when the movement of the parts instead of being conjunct, should be disjunct, the discordance not the less exists.
Parallel fifths by disjunct motion
Here is one of these defects arising from “direct movement”, which it was previously promised should be pointed out.
Consecutive 7) fifths have been, and still are, tolerated in “contrary movement”, because if they are of the same kind, this movement causes them to change their nature.
Contrary fifths – Unacceptable in two-part counterpoint
In this example it will be seen that one is a twelfth, and the other a fifth, which changes their nature. Nevertheless, it is forbidden to use this permission in two-part counterpoint, particularly note against note; this method is tolerated in middle parts, when composing for four voices, where there is difficulty in making the parts flow well.
The pupil may meet in works of free composition – as operas, symphonies, etc. – with consecutive 8) fifths; but these licenses are only to be tolerated in this style of composition.
Rule 5
It is prohibited to pass to a perfect concord 9) by direct movement, excepting when one of the two proceeds by semitone 10). This exception is tolerated.
Prohibited movements:
The movements in Example 14 are prohibited, because, supposing the distances formed by the intervals are filled by notes of inferior value ascending or descending, there would be either two fifths or two octaves – called two concealed 11) fifths or octaves:–
Observation – This rule, at first sight, seems ill-founded; because, the intervention of crotchets 12) not being written down by the composer, the two fifths or two octaves do not perceptively exist. But the singer may add these crotchets 13); and in that case, the two fifths or two octaves are clearly heard. The ancient composers, in order to evade the objectionable point which would arise from the inconsiderate license that the singer might take, forbade going to a perfect concord 14) by direct movement. The rule, therefore, to prefer contrary movement, is excellent, because it prevents falling into the defect – hidden though it be – of which direct movement is the cause. This rule, also, indicates yet another objectionable point occasioned by direct movement.
As to the tolerated movement, instanced in Example 15, there the case is different; inasmuch as by filling up with crotchets 15) the spaces marked by the intervals, there result, it is true, two fifths, but one is imperfect, the other perfect. 16)
Example 15 with crotchets
These two fifths are tolerated, because they are not of the same nature, and because the discord 17) of which we have spoken, arising from perfect fifths in succession, does not take place in this ease. The old composers avoided this method in two-part counterpoint; it was only in composition for several voices that they availed themselves of it in one of the middle parts, when they desired to escape from some perplexing point.
Rule 6
All movement should be either diatonic or natural, in regard to melody; and conjunct movement better suits the style of strict counterpoint than disjunct movement. Accordingly, movement of the major and minor second, of the major and minor third, of the perfect fourth, of the perfect fifth, of the minor sixth, and of the octave, are permitted either ascending or descending. The movement of the superfluous 18) fourth, or tritone, of the imperfect fifth, and of the major and minor seventh, are expressly prohibited either ascending or descending.
Observation – This rule is a very wise one; and the ancient masters were all the more judicious in observing it, since they wrote for voices alone, without accompaniment. They thus obtained an easy and correct melody, where prohibited movement would have rendered it difficult of intonation. Nevertheless, this rule has been much deviated from, in modern composition.
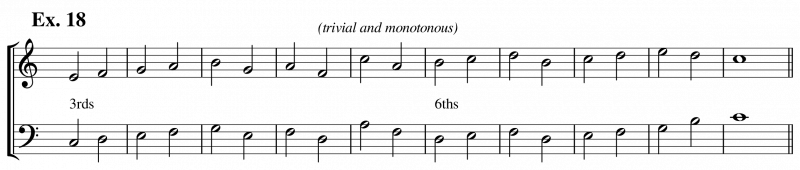
With regard to movement which should be employed in the case of one part respectively with another, it is, as has been already said, contrary movement that should be preferred to oblique movement, and this latter, to direct movement. The last should be very seldom used; for even when all the rules are observed which have been laid down to evade the objectionable point would be incurred – not positively contrary to rule, but contrary to good taste, good style, and the diversity of concords 19); since by this movement, there would be a long succession of either thirds or sixths – producing an effect both trivial and monotonous.
This example offers throughout the same concord 20), the same movement, and consequently the same unvaried effect.
Observation – As many as three thirds, or three sixths in succession, at the utmost, may be used; but to go beyond that number would be a wilful committal of pre-stated error.
Rule 7
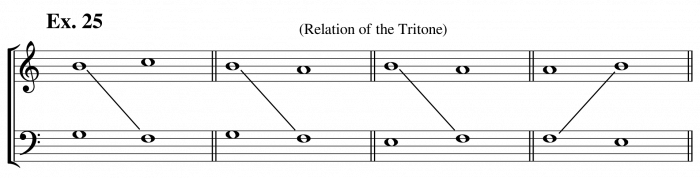
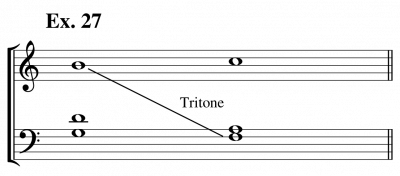
False relation of the octave, and of the tritone, between the parts, should be avoided; these two relations are harsh to the ear, – especially that of the octave.
Observation – Relation signifies the immediate affinity existing mutually between two sounds, successive or simultaneous. This affinity is considered according to the nature of the interval formed by the two sounds, so that the relation shall be true when the interval is true; it is false when there is alteration by excess or diminution. Among false relations, those only are included, in harmony, of which the two sounds do not equally belong to the key in which they occur. The diminished octave, or the superfluous 21) octave, is a false relation in melody as in harmony, however they may be used. The disagreeable effect if produces may be mitigated, but not entirely destroyed. The employment of this movement is therefore prohibited in melody:–
False relations of the diminished octave and the augmented octave
In harmony, the use of these octaves struck simultaneously, and held down for some time, is inadmissible.
Nevertheless, there are modern composers who have thought fit to employ it, thus:–
In this case they consider the C-flat and the C-sharp but as passing alterations, and as notes of little value struck in the unaccented part of the bar.
It it a very great license, which is only just to be tolerated in a style of composition of the freest kind, but which should be rejected altogether in strict counterpoint. There exists another case, in which the false relation of the octave in harmony may be hazarded, between two different chords, as thus:–
The C-natural in Example 22, introduced with the first chord in the upper part, forms a discord 22) with the C-sharp introduced into the second chord in the lower part. If the sense of hearing be consulted on the subject, it will be agreed that nothing can destroy, in this case, the impression which the ear has received from the sound of the C-natural; because it still lasts while the sound of the C-sharp is being struck, which produces nearly the same effect as if these two sounds were simultaneous. If reason be consulted, in its turn, it will be decided that the discord 23) formed by these two sounds originates in their irrelevance, and from the false affinity that exists between them, since C-natural and C-sharp each belong to two different keys, and the chords which severally contain them, cannot follow one another in the succession in which they are here placed; unless other intermediate and relative chords, by linking them together, be made to obviate the false relation. What has just been said respecting Example 22 is equally applicable to Example 23.
In order to render the effect less harsh in the succession of these two chords – as it is impossible to destroy it entirely – a softening expedient of protraction must be found without employing other chords. The means are simple; it must be so managed, that the part which has struck the C-natural must cause the altered C to be heard.
By these simple means, and other expedients, somewhat similar, the unpleasant impression may be in a measure mitigated or rendered scarcely perceptible, because the ear not being hurt so immediately in this case as in the other, lends itself by degrees to endure the impression of the false relation. Nevertheless, in a study of modern strict counterpoint, this chromatic movement should be as much as possible avoided.
The tritone is always, in melody, a false relation; besides being a prohibited movement (see Rule 6).
This interval produces also a false relation in harmony, especially in two-part counterpoint of the first order, when these parts are disposed in such a way that this interval is visibly present.
This interval is visibly present, when the two sounds of which it is composed are to be heard one after the other in the two parts, and when the chords which contain them cannot belong to the same key, either by their nature or by the manner in which they succeed each other:–
Care should be taken to avoid entirely such kind of relation, in two-part counterpoint more especially; and if they are not to be avoided, endeavour should at least be made so as to mask them, in disposing the part which forms the counterpoint, as that one of the two sounds which constitute the tritone may be suppressed, whether a change be made, or the same chords be preserved:–
By the aid of these corrections, the relation is partly, or entirely veiled. In the other orders of counterpoint, as will be seen, it is easier, than in this one, to avoid the false relations of tritone.
It now remains to be demonstrated, how and why the tritone is a false relation in harmony. What I am about to state, applies equally to two-part counterpoint, as to that in several parts, and I here subjoin this demonstration in order not to have occasion hereafter for mentioning it with so much detail.
In order therefore to explain the course of this false relation, I take the major common chord of G, which I cause to be immediately succeeded by that of F:–
The succession of these two chords instantly generates the false relation of tritone. Firstly, because the first chord, supposing it to be considered as belonging to the key of C, naturally tends to proceed to the tonic or to the relative minor of A, and not to the sub-dominant. Secondly, supposing, on the contrary, that this same chord belongs to the key of G, the chord of F-natural which follows, becomes alien to it, since it would be requisite that the F should be F-sharp, in order that the analogy between these two chords should exist; and moreover this F-sharp ought to carry the chord of the sixth 24). Thirdly, by the same process of reasoning, if the second chord be considered as belonging to the key of C, or to the key of F, in the former hypotheses, it would require to be followed and not preceded by the chord of G, and in the latter case, the B-natural of the chord of G, becomes necessarily and evidently alien to it, since by analogy this B should be flat. Thus then the F and B being in open contradiction, the one by the other, and the one with the other, the relation which results between them is false.
Consequently, all successions of chords, of which one contains an F, and the other a B, and vice versa, indisputably bring about the false relation of tritone. Here is a succession of chords, which always present this relation, and which accordingly produce a very harsh effect:–
Rule 8
Except at the first bar, and the last, imperfect concords 25) should always be introduced, in the course of composition, in preference to perfect ones. The object of this rule is to produce harmony by means of imperfect concords 26), which are more harmonious than the others. Nevertheless, the employment of may imperfect concords 27) of the same denomination would lead to the abuse pointed out in Rule 6, which should be carefully avoided. The composer should know how to intermingle with taste and discernment perfect and imperfect concords 28), in order to give harmony to the counterpoint.
Two completed examples of first-species counterpoint
These examples are in conformity with the rules of strict counterpoint of the first order. Imperfect concords 29) are employed with variety, and more frequently than perfect concords 30). Direct, contrary, and oblique movement, are judiciously treated; the false relation of tritone is avoided; and the melody progresses through, diatonically, with ease and elegance.
Observation – In order to put in practice all the rules above cited, the pupil will receive from his instructor a cantus firmus 31), which he should first place in the bass, and upon which he should compose as many different melodies as he can invent, – always employing alternately Soprano, Alto, and Tenor voices. Then he must place this cantus firmus in the upper part, and compose to it several Basses.
This cantus firmus, which the pupil receives from his instructor, is also called the given-subject, or plain-song; the part composed by the pupil is termed Counterpoint 32).
There will be found at the end of this treatise, several different canti firmi, for each of the orders of counterpoint, which will give the pupil an opportunity of employing all the resources of counterpoint.
When placing the cantus firmus in the upper part, the pupil should employ the voice best adapted to this plain-song; and sometimes he will find himself compelled to transpose its key, in order that he may employ the different voices without transgressing the limits of their compass.
As the two last bars of the cantus firmus should always progress from the second of the key to the tonic – for example, for the key of C, as RE to DO. The penultimate bar which forms the counterpoint must always be a major sixth, and the last bar an octave, provided the cantus firmus be in the Bass; and if it be in the upper part, the penultimate bar will be a minor third, and the last bar an octave. As thus:–
Before concluding the first order of counterpoint, a word must be said respecting modulations; and the observations upon this head will be applicable to all kinds of strict counterpoint.
Modulation should never be made, in any piece whatever, excepting into those keys, the tonics of which form part of the scale constituting the original key.
Supposing the scale of C is the original key, we can only modulate into G major, into A (the relative minor), into F major, and into D minor. Moreover, we must only touch in passing, the key of F, because it weakens the principal key on account of the B-flat which destroys the leading-tone. The same mode of treatment must be pursued with the key of D minor, for the same reason as the key of F, more particularly as it destroys the tonic, by the C-sharp which is the leading-tone of this key. We may also modulate into E minor, but not remain in that key, still less than in the two keys above-mentioned, on account of the F-sharp and the D-sharp which are introduced by it. The key of B is proscribed, because it has no perfect fifth.
Supposing now, the scale to be A minor, the relative of C. We may first modulate into C major, and touch, in passing, the keys of F major, and of D minor; that of E minor may be sustained. They key of B is proscribed in this original key, for the same reason as in the original key of C.
All these modulations are natural and analogous to the principal or original key. It is experience and study, which will enable the pupil to introduce these several keys, in a judicious and agreeable manner.